| 1 | x−2 | |||
limx→0 | − | |||
| x2 | x3−x |
| 4 | 1 | |||
limx→1 | − | |||
| 1−x | (x−1)2 |
| 1 | x−2 | (x2−1) − x*(x−2) | 2x + 1 | |||||
1. | − | = | = − | |||||
| x2 | x3−x | x2(x2−1) | x(x2−1) |
| 4 | 1 | 4(x−1) | 4 | 4x | ||||||
2. | − | = − | − | = − | ||||||
| 1−x | (x−1)2 | (x−1)2 | (x−1)2 | (x−1)2 |
| 1 | x−2 | 2x−1 | ||||
W 1 wychodzi mi | − | = | ||||
| x2 | x3−x | x2(x2−1) |
| −1 | ||
Liczac granice lewostronna | =−nieskonczonosc | |
| 0+ |
| −1 | ||
prawostronna | =−nieskonczonosc | |
| 0+ |
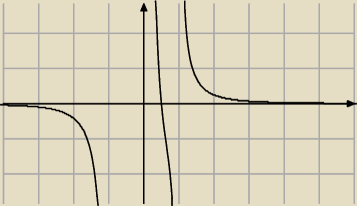 lewostronna (−∞)
lewostronna (−∞)
| −1 | ||
prawostronna (+∞) bo masz symbol : [ | } | |
| 0+*(−1) |
| 5^2 | 52 |
| 2^{10} | 210 |
| a_2 | a2 |
| a_{25} | a25 |
| p{2} | √2 |
| p{81} | √81 |
| Kliknij po więcej przykładów | |
|---|---|
| Twój nick | |