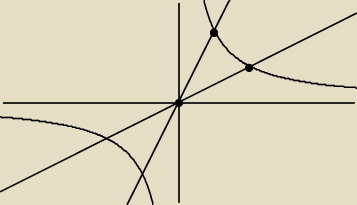
. Natalka: Mógłby ktoś wytłumaczyć? Narysuj i wyznacz pola poniższych zbiorów

	1		1
a) A = {(x,y)∊R2: 0 ≤ y ≤		, y ≤ 2x, y ≥		x
	x		2

Figura określona przez te warunki to ten 'trojkat' w pierwszej cwiartce. Nauczyciel podzielił go na dwie części, chociaż nie bardzo wiem jak (mam niedokladnie narysowane).

	1
PUNKT P1 (przecięcie y=2x i y=
	x

	1		√2		√2
2x=		⇒ x =		} lub x= −
	x		2		2

Natalka: zaraz dopiszę resztę (niechący kliknęłam "wyślij") undefined

Natalka: P2:

1		1
	x =		⇒ x=√2 lub x=−√2
2		x

POLE S1:

	√2		1		3
∫ indeks górny		indeks dolny 0 z (2x−		x)dx =		j2
	2		2		8

	√2
nie bardzo wiem dlaczego zostały tutaj wybrane przy oznaczeniu całki		} i 0
	2

	1
oraz skąd proste y=2x i y=		x
	2

Natalka:

	√2		1		1		3
S2 to całka o indeksie górnym √2 i dolnym		z (		−		x)dx = ln2 −
	2		x		2		8

Na końcu S1+S2 = ln2

Natalka: nie wiem tylko kiedy brać jakie oznaczenia całek i jakie proste.

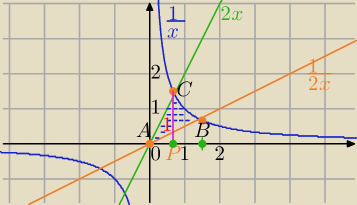Mila: Musisz trójkąt , tak podzielić , abyś miała obszary normalne względem osi OX, ( albo OY) C:

	1
2x=		, x>0
	x

2x²=1

	√2
x=
	2

	√2
y=2*		=√2
	2

	√2
C=(		,√2)
	2

	√2
P=(		,0)
	2

B:

1		1
	x=		, x>0
2		x

1
	x2=1
2

x²=2 x=√2

	√2
y=
	2

	√2
B=(√2,		)
	2

	√2
P1−Całka (górna funkcja − dolna funkcja, tak zmienia się y), granice od x=0 do x=
	2

	1		3		3
P1=x=0∫x=√2/2(2x−		x) dx =∫0∫√2/2(		x) dx=[		x2]0√2/2=
	2		2		4

	3		√2		3		1		3
=		(		)2−0=		*		=
	4		2		4		2		8

	√2
P2 − całka górna funkcja − dolna funkcja , granice x=		do x=√2
	2

	1		1		1
P2=x=√2/2∫x=√2(		−		x) dx=[lnx−		x2]x=√2/2x=√2=
	x		2		4

	1		√2		1		1
=ln√2−		*2−ln(		)+		*		=
	4		2		4		2

	1		1		3
=ln√2−		−ln√2+ln(2)+		=ln(2)−
	2		8		8

S=ln(2)

Natalka : Dziękuję Mila!

Mila: